



Next: 2.2 A collection of
Up: 2 Equations
Previous: 2 Equations
Contents
Index
So far, this section demonstrates only how nicely LATEX can display formulae...
The 3D hydrodynamics equations,
including source terms due to gravity, are
the mass conservation equation
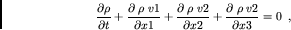 |
(1) |
the momentum equation
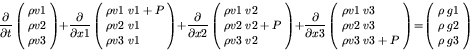 |
(2) |
and the energy equation
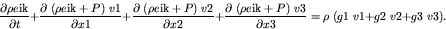 |
(3) |
In addition, there are equations for the 3D tensor viscosity and the non-local radiation transport.