2.2.1 Simple ODE: discretization

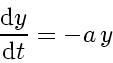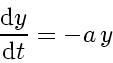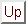
The simple ordinary differential equation (ODE)

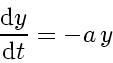$$\frac{\mathrm{d} y}{\mathrm{d} t} = - a y$$ (71)

with initial value

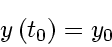$$y \left( t_0 \right) = y_0$$ (72)

is of first order and linear with constant coefficient $a$. It has the obvious solution

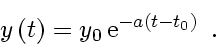$$y \left( t \right) = y_0 \mathrm{e}^{- a \left( t - t_0 \right)} \enspace .$$ (73)

For discrete time-steps

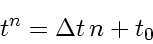$$t^{n} = \Delta t n + t_0$$ (74)

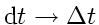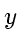the straight-forward replacement $\mathrm{d} t \rightarrow \Delta t$ gives the most simple discretization (explicit Euler scheme: approximation of $y$ by a piecewise linear curve)

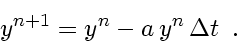$$y^{n+1} = y^{n} - a y^{n} \Delta t \enspace .$$ (75)