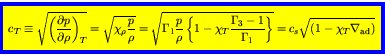Next: 2.2.5 Ideal gas with
Up: 2.2 A collection of
Previous: 2.2.3 CO5BOLD equation of
Contents
Index
First, the missing derivative
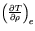 can be found from the relation:
can be found from the relation:
 |
(22) |
which is obtained from the equality of the mixed derivatives in
Eq.4, written as:
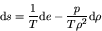 |
(23) |
Then
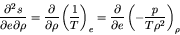 |
(24) |
First adiabatic exponent:
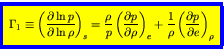 |
(25) |
This relation is obtained by combining Eq.4 with the identity
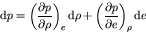 |
(26) |
The adiabatic sound speed
is then obtained as
 |
(27) |
Third adiabatic exponent:
 |
(28) |
This relation is obtained by combining Eq.4 with the identity
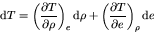 |
(29) |
and then using Eq.22.
Adiabatic temperature gradient:
 |
(30) |
since
 |
(31) |
Adiabatic energy changes:
 |
(32) |
or
 |
(33) |
We define the coefficients 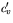 and
and 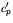 through the
relation
through the
relation
 |
(34) |
Entropy change at constant density:
 |
(35) |
This relation is obtained from the equality of the mixed derivatives in
Eq.4 together with Eq.28.
Entropy change at constant pressure:
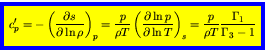 |
(36) |
This relation is obtained from the equality of the mixed derivatives in
Eq.5 together with Eq.30.
Specific heat at constant density:
 |
(37) |
To derive the specific heat at constant pressure, we start from the relation
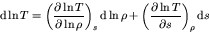 |
(38) |
from which we get
 |
(39) |
Using Eqs. 28 and 37, we obtain
 |
(40) |
Now
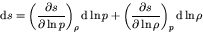 |
(41) |
or
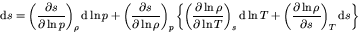 |
(42) |
hence
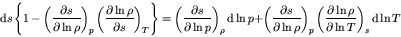 |
(43) |
and finally
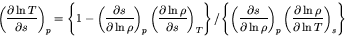 |
(44) |
or
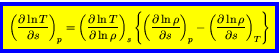 |
(45) |
Using Eqs. 11, 28, 36, 40, we finally
obtain the relation for the specific heat at constant pressure:
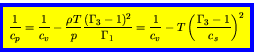 |
(46) |
Alternatively, 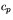 can be obtained from Eq.16
can be obtained from Eq.16
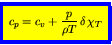 |
(47) |
or from
 |
(48) |
once  and
and 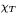 are known (see below).
are known (see below).
We can now express the thermodynamic coefficients provided by CO5BOLD
in terms of  ,
,  ,
, 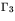 , and
, and
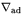 :
:
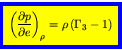 |
(49) |
 |
(50) |
 |
(51) |
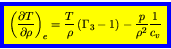 |
(52) |
 |
(53) |
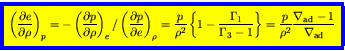 |
(54) |
We consider again Eq.23, replacing 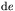 by
by
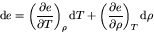 |
(55) |
so
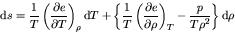 |
(56) |
The requirement that the mixed derivatives must be equal then yields
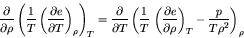 |
(57) |
or
 |
(58) |
Finally,
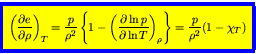 |
(59) |
Comparison with Eq.53 implies
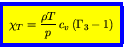 |
(60) |
Similarly, replacing 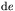 by
by
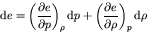 |
(61) |
in Eq.23, we get
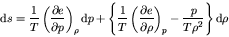 |
(62) |
and the requirement that the mixed derivatives must be equal then yields
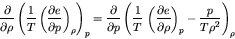 |
(63) |
or
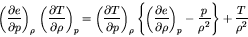 |
(64) |
or
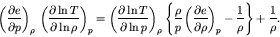 |
(65) |
Since
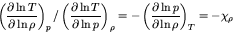 |
(66) |
we finally obtain, using Eqs.13, 49 and 54,
 |
(67) |
and
 |
(68) |
The isothermal sound speed
is then obtained as
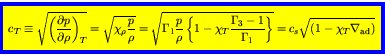 |
(69) |





Next: 2.2.5 Ideal gas with
Up: 2.2 A collection of
Previous: 2.2.3 CO5BOLD equation of
Contents
Index

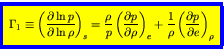

![]() and
and ![]() through the
relation
through the
relation
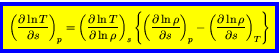
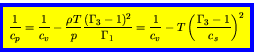
![]() ,
, ![]() ,
, ![]() , and
, and
![]() :
:

![]() by
by
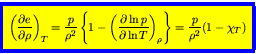
![]() by
by