3.1.3 Compression waves and shocks

A compression wave with

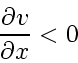$$\frac{\partial v}{\partial x} < 0$$ (163)

steepens with time and characteristic curves can cross: multiple-valued solution?

• The viscous Burgers' equation (158) is a parabolic PDE and has a unique solution for all times $t > 0$.
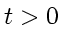• Vanishing viscosity: We look for a solution of the inviscid Burgers' equation that is a solution of the viscous Burgers' equation in the limit $\epsilon \rightarrow 0$.
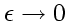• Instead of a multiple-valued solution we get a discontinuity where the characteristics end.
• Discontinuities (shocks) are unavoidable for $\frac{\partial v}{\partial x} < 0$.
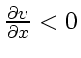• Characteristics run into a shock from both sides.
• Discontinuities should be allowed in the initial conditions.
• We need to find weak solutions.
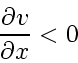
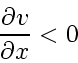
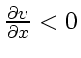